HJM Framework - Interest Rate Term Structure Models

Introduces HJM (Heath Jarrow Morton) and explain key concepts. Also derives the drift condition under the risk neutral measure, forward measure, and terminal forward. And discusses few specification of volatility such as deterministic volatility and separable volatility which make the process Gaussian and Markovian, respectively. Here is the outline of the content by timeline:

0:11/19:57: Explains visually what is being modelled by the HJM framework
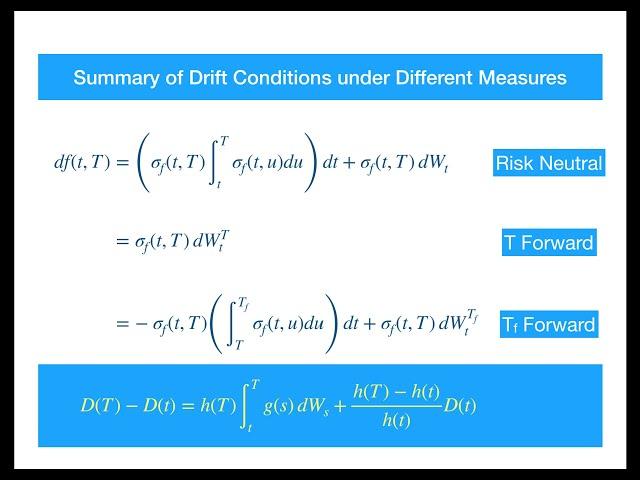
1:53/19:57: Derive the HJM drift condition under the Risk neutral measure
6:18/19:57: Derive the HJM drift condition under the T-Forward measure
11:16/19:57:Derive the HJM drift condition under the Terminal Forward measure
13:51/19:57: Highlights the importance of the Volatility or diffusion term in the HJM
14:23/19:57: Explains what specification would make the HJM Gaussian, and Markovian
17:42/19:57: Explains why log-normal or geometric brownian SDE won’t work in the HJM framework

Тэги:

#HJM #Heath_Jarrow_Morton #Terminal_Forward #Drift_Condition #T-Forward_Measure #Forward_Measure #HJM_Drift_Condition #Separable_Volatility #Gaussian #Markovian #Markov_Process #HJM_Framework #Heath-Jarrow-Morton_(HJM)_Framework #Log-Normal_HJM

Скачать видео